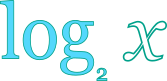Jump to
-  Calculator
-  Definitions
Table of Contents
-  Calculator
-  Definitions
-  General
-  Properties
Share this
See also
Evaluate log10x
Evaluate ln(x)
Evaluate lognx
Evaluate n-th power
All evaluation tools

Binary logarithm calculator

- By Dr. Minas E. Lemonis, PhD - Updated: March 3, 2019
Home > Evaluation > Binary logarithm

This tool evaluates the binary logarithm of a number: \log_{2}{x} . Enter the argument x below.

x =
icon

Result:
\log_{2} {x} =
shape details

ADVERTISEMENT

Table of Contents
-  Calculator
-  Definitions
-  General
-  Properties
Share this

Definitions

General

The binary logarithm of a number x, is a power p so that 2p= x. In modern notation binary logarithm is written as \log_{2}{x} . Any positive real number has a binary logarithm. The binary logarithm of 1 is 0, while the binary logarithm of 0 approaches negative infinity.

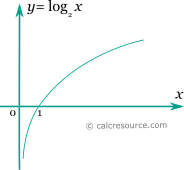
The graph of the binary logarithm function is shown in the figure below. It is a monotonic function.

loge-graph

Properties

The derivative of the binary logarithm function is:

\left(\log_{2} x\right)' = \frac{1}{x}\frac{1}{\ln{2}} \approx \frac{1.44270}{x}

The integral of the binary logarithm function is given by:

\int \log_{2} x\, \mathrm{d}x = \frac{x\ln{x}-x}{\ln{2}} +C

See also
Evaluate log10x
Evaluate ln(x)
Evaluate lognx
Evaluate n-th power
All evaluation tools