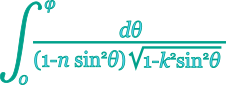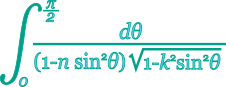ADVERTISEMENT
About Elliptic Integrals of the Third Kind
Definitions
The incomplete elliptic integral of the third kind is defined as:
where k is the elliptic modulus, with , and n is a parameter called elliptic characteristic, that can take any real value. Variable is the Jacobi's amplitude.
For n=0, the elliptic integral of the third kind is identical to the respective integral of the first kind:
The complete elliptic integral of the third kind is defined as:
For n=1, the complete elliptic integral of the third kind becomes infinite for any k:
Values
In the following table the values of the complete elliptic integral of the third kind are shown for a range of k and n values:
| k | Π(k,-10) | Π(k,-5) | Π(k,-2) | Π(k,-1) | Π(k,0) | Π(k,1) | Π(k,2) | Π(k,5) | Π(k,10) |
|---|---|---|---|---|---|---|---|---|---|
| -1 | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ |
| -0.999 | 0.7719 | 1.177 | 1.948 | 2.64 | 4.496 | ∞ | -3.242 | -0.8537 | -0.384 |
| -0.99 | 0.6666 | 0.9849 | 1.564 | 2.066 | 3.357 | ∞ | -2.066 | -0.5619 | -0.2545 |
| -0.98 | 0.6347 | 0.9266 | 1.449 | 1.894 | 3.021 | ∞ | -1.701 | -0.472 | -0.2147 |
| -0.97 | 0.6159 | 0.8925 | 1.382 | 1.795 | 2.828 | ∞ | -1.484 | -0.4186 | -0.191 |
| -0.96 | 0.6025 | 0.8682 | 1.334 | 1.725 | 2.693 | ∞ | -1.329 | -0.3802 | -0.1741 |
| -0.95 | 0.5921 | 0.8494 | 1.298 | 1.671 | 2.59 | ∞ | -1.208 | -0.3502 | -0.1608 |
| -0.9 | 0.5597 | 0.7913 | 1.186 | 1.507 | 2.281 | ∞ | -0.8352 | -0.2554 | -0.1187 |
| -0.8 | 0.5276 | 0.7346 | 1.078 | 1.352 | 1.995 | ∞ | -0.4841 | -0.16 | -0.07571 |
| -0.7 | 0.5096 | 0.7031 | 1.019 | 1.268 | 1.846 | ∞ | -0.3036 | -0.1061 | -0.05094 |
| -0.6 | 0.4977 | 0.6824 | 0.9813 | 1.215 | 1.751 | ∞ | -0.1933 | -0.07043 | -0.03421 |
| -0.5 | 0.4892 | 0.6679 | 0.9548 | 1.177 | 1.686 | ∞ | -0.1207 | -0.04539 | -0.02225 |
| -0.4 | 0.4831 | 0.6574 | 0.9359 | 1.151 | 1.64 | ∞ | -0.07137 | -0.02748 | -0.01357 |
| -0.3 | 0.4788 | 0.65 | 0.9226 | 1.132 | 1.608 | ∞ | -0.0379 | -0.01484 | -0.007372 |
| -0.2 | 0.4758 | 0.6451 | 0.9137 | 1.12 | 1.587 | ∞ | -0.01619 | -0.006418 | -0.0032 |
| -0.1 | 0.4742 | 0.6422 | 0.9086 | 1.113 | 1.575 | ∞ | -0.003957 | -0.001579 | -0.000789 |
| 0 | 0.4736 | 0.6413 | 0.9069 | 1.111 | 1.571 | ∞ | 0 | 0 | 0 |
| 0.1 | 0.4742 | 0.6422 | 0.9086 | 1.113 | 1.575 | ∞ | -0.003957 | -0.001579 | -0.000789 |
| 0.2 | 0.4758 | 0.6451 | 0.9137 | 1.12 | 1.587 | ∞ | -0.01619 | -0.006418 | -0.0032 |
| 0.3 | 0.4788 | 0.65 | 0.9226 | 1.132 | 1.608 | ∞ | -0.0379 | -0.01484 | -0.007372 |
| 0.4 | 0.4831 | 0.6574 | 0.9359 | 1.151 | 1.64 | ∞ | -0.07137 | -0.02748 | -0.01357 |
| 0.5 | 0.4892 | 0.6679 | 0.9548 | 1.177 | 1.686 | ∞ | -0.1207 | -0.04539 | -0.02225 |
| 0.6 | 0.4977 | 0.6824 | 0.9813 | 1.215 | 1.751 | ∞ | -0.1933 | -0.07043 | -0.03421 |
| 0.7 | 0.5096 | 0.7031 | 1.019 | 1.268 | 1.846 | ∞ | -0.3036 | -0.1061 | -0.05094 |
| 0.8 | 0.5276 | 0.7346 | 1.078 | 1.352 | 1.995 | ∞ | -0.4841 | -0.16 | -0.07571 |
| 0.9 | 0.5597 | 0.7913 | 1.186 | 1.507 | 2.281 | ∞ | -0.8352 | -0.2554 | -0.1187 |
| 0.95 | 0.5921 | 0.8494 | 1.298 | 1.671 | 2.59 | ∞ | -1.208 | -0.3502 | -0.1608 |
| 0.96 | 0.6025 | 0.8682 | 1.334 | 1.725 | 2.693 | ∞ | -1.329 | -0.3802 | -0.1741 |
| 0.97 | 0.6159 | 0.8925 | 1.382 | 1.795 | 2.828 | ∞ | -1.484 | -0.4186 | -0.191 |
| 0.98 | 0.6347 | 0.9266 | 1.449 | 1.894 | 3.021 | ∞ | -1.701 | -0.472 | -0.2147 |
| 0.99 | 0.6666 | 0.9849 | 1.564 | 2.066 | 3.357 | ∞ | -2.066 | -0.5619 | -0.2545 |
| 0.999 | 0.7719 | 1.177 | 1.948 | 2.64 | 4.496 | ∞ | -3.242 | -0.8537 | -0.384 |
| 1 | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ | ∞ |
The xy plots of the complete integrals of the third kind for various values of characteristic n are depicted in the following figure:
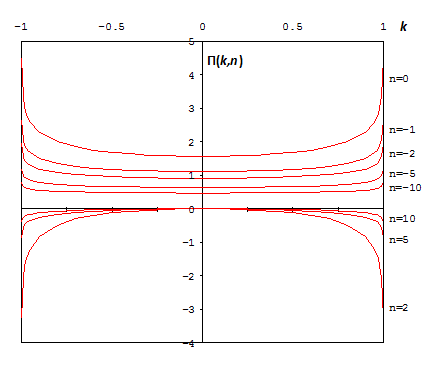
The curve for n=1 is not drawn because, the integral becomes infinite. Also, for n>1 it is .
In the following table the values of the incomplete elliptic integral of the third kind are shown for n=0 and a range of k and φ values. These values are identical to the respective ones of the incomplete elliptic integral of the first kind because .
| k | Π(30°,k,0) | Π(45°,k,0) | Π(60°,k,0) | Π(90°,k,0) | Π(135°,k,0) | Π(225°,k,0) | Π(270°,k,0) | Π(315°,k,0) |
|---|---|---|---|---|---|---|---|---|
| -1 | 0.5493 | 0.8814 | 1.317 | ∞ | 0.8814 | -0.8814 | -∞ | -0.8814 |
| -0.999 | 0.5492 | 0.8811 | 1.316 | 4.496 | 0.8811 | -0.8811 | -4.496 | -0.8811 |
| -0.99 | 0.5487 | 0.8787 | 1.307 | 3.357 | 0.8787 | -0.8787 | -3.357 | -0.8787 |
| -0.9 | 0.5439 | 0.8579 | 1.233 | 2.281 | 0.8579 | -0.8579 | -2.281 | -0.8579 |
| -0.8 | 0.5393 | 0.8396 | 1.179 | 1.995 | 0.8396 | -0.8396 | -1.995 | -0.8396 |
| -0.6 | 0.5321 | 0.8135 | 1.111 | 1.751 | 0.8135 | -0.8135 | -1.751 | -0.8135 |
| -0.4 | 0.5273 | 0.7973 | 1.073 | 1.64 | 0.7973 | -0.7973 | -1.64 | -0.7973 |
| -0.2 | 0.5245 | 0.7883 | 1.053 | 1.587 | 0.7883 | -0.7883 | -1.587 | -0.7883 |
| 0 | 0.5236 | 0.7854 | 1.047 | 1.571 | 0.7854 | -0.7854 | -1.571 | -0.7854 |
| 0.2 | 0.5245 | 0.7883 | 1.053 | 1.587 | 0.7883 | -0.7883 | -1.587 | -0.7883 |
| 0.4 | 0.5273 | 0.7973 | 1.073 | 1.64 | 0.7973 | -0.7973 | -1.64 | -0.7973 |
| 0.6 | 0.5321 | 0.8135 | 1.111 | 1.751 | 0.8135 | -0.8135 | -1.751 | -0.8135 |
| 0.8 | 0.5393 | 0.8396 | 1.179 | 1.995 | 0.8396 | -0.8396 | -1.995 | -0.8396 |
| 0.9 | 0.5439 | 0.8579 | 1.233 | 2.281 | 0.8579 | -0.8579 | -2.281 | -0.8579 |
| 0.99 | 0.5487 | 0.8787 | 1.307 | 3.357 | 0.8787 | -0.8787 | -3.357 | -0.8787 |
| 0.999 | 0.5492 | 0.8811 | 1.316 | 4.496 | 0.8811 | -0.8811 | -4.496 | -0.8811 |
| 1 | 0.5493 | 0.8814 | 1.317 | ∞ | 0.8814 | -0.8814 | -∞ | -0.8814 |
The xy plots of the incomplete integrals of the third kind for characteristic n=0 are depicted in the following figure. The curves are identical to the respective ones of the first elliptic integral.
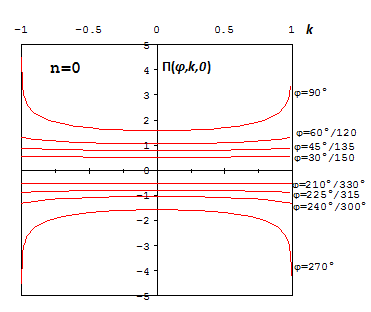
In the following table the values of the incomplete elliptic integral of the third kind are shown for n=1 and a range of k and φ values:
| k | Π(30°,k,1) | Π(45°,k,1) | Π(60°,k,1) | Π(90°,k,1) | Π(135°,k,1) | Π(225°,k,1) | Π(270°,k,1) | Π(315°,k,1) |
|---|---|---|---|---|---|---|---|---|
| -1 | 0.608 | 1.148 | 2.391 | ∞ | 1.148 | -1.148 | -∞ | -1.148 |
| -0.999 | 0.6079 | 1.147 | 2.388 | ∞ | 1.147 | -1.147 | -∞ | -1.147 |
| -0.99 | 0.6073 | 1.144 | 2.363 | ∞ | 1.144 | -1.144 | -∞ | -1.144 |
| -0.9 | 0.6015 | 1.111 | 2.174 | ∞ | 1.111 | -1.111 | -∞ | -1.111 |
| -0.8 | 0.596 | 1.083 | 2.039 | ∞ | 1.083 | -1.083 | -∞ | -1.083 |
| -0.6 | 0.5875 | 1.043 | 1.878 | ∞ | 1.043 | -1.043 | -∞ | -1.043 |
| -0.4 | 0.5817 | 1.018 | 1.791 | ∞ | 1.018 | -1.018 | -∞ | -1.018 |
| -0.2 | 0.5784 | 1.004 | 1.746 | ∞ | 1.004 | -1.004 | -∞ | -1.004 |
| 0 | 0.5774 | 1 | 1.732 | ∞ | 1 | -1 | -∞ | -1 |
| 0.2 | 0.5784 | 1.004 | 1.746 | ∞ | 1.004 | -1.004 | -∞ | -1.004 |
| 0.4 | 0.5817 | 1.018 | 1.791 | ∞ | 1.018 | -1.018 | -∞ | -1.018 |
| 0.6 | 0.5875 | 1.043 | 1.878 | ∞ | 1.043 | -1.043 | -∞ | -1.043 |
| 0.8 | 0.596 | 1.083 | 2.039 | ∞ | 1.083 | -1.083 | -∞ | -1.083 |
| 0.9 | 0.6015 | 1.111 | 2.174 | ∞ | 1.111 | -1.111 | -∞ | -1.111 |
| 0.99 | 0.6073 | 1.144 | 2.363 | ∞ | 1.144 | -1.144 | -∞ | -1.144 |
| 0.999 | 0.6079 | 1.147 | 2.388 | ∞ | 1.147 | -1.147 | -∞ | -1.147 |
| 1 | 0.608 | 1.148 | 2.391 | ∞ | 1.148 | -1.148 | -∞ | -1.148 |
The xy plots of the incomplete integrals of the third kind for characteristic n=1 are depicted in the following figure.
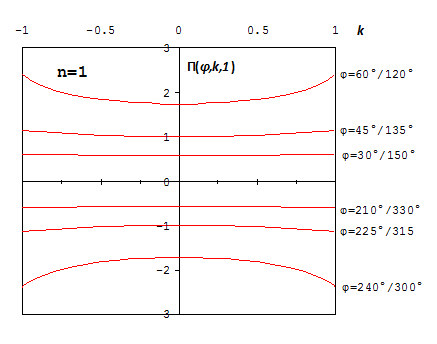
In the following table the values of the incomplete elliptic integral of the third kind are shown for n=-1 and a range of k and φ values:
| k | Π(30°,k,-1) | Π(45°,k,-1) | Π(60°,k,-1) | Π(90°,k,-1) | Π(135°,k,-1) | Π(225°,k,-1) | Π(270°,k,-1) | Π(315°,k,-1) |
|---|---|---|---|---|---|---|---|---|
| -1 | 0.5065 | 0.7484 | 1.015 | ∞ | 0.7484 | -0.7484 | -∞ | -0.7484 |
| -0.999 | 0.5064 | 0.7482 | 1.015 | 2.64 | 0.7482 | -0.7482 | -2.64 | -0.7482 |
| -0.99 | 0.506 | 0.7465 | 1.009 | 2.066 | 0.7465 | -0.7465 | -2.066 | -0.7465 |
| -0.9 | 0.5018 | 0.7308 | 0.9613 | 1.507 | 0.7308 | -0.7308 | -1.507 | -0.7308 |
| -0.8 | 0.4978 | 0.7169 | 0.9254 | 1.352 | 0.7169 | -0.7169 | -1.352 | -0.7169 |
| -0.6 | 0.4916 | 0.6971 | 0.8802 | 1.215 | 0.6971 | -0.6971 | -1.215 | -0.6971 |
| -0.4 | 0.4874 | 0.6846 | 0.8545 | 1.151 | 0.6846 | -0.6846 | -1.151 | -0.6846 |
| -0.2 | 0.485 | 0.6777 | 0.8409 | 1.12 | 0.6777 | -0.6777 | -1.12 | -0.6777 |
| 0 | 0.4842 | 0.6755 | 0.8366 | 1.111 | 0.6755 | -0.6755 | -1.111 | -0.6755 |
| 0.2 | 0.485 | 0.6777 | 0.8409 | 1.12 | 0.6777 | -0.6777 | -1.12 | -0.6777 |
| 0.4 | 0.4874 | 0.6846 | 0.8545 | 1.151 | 0.6846 | -0.6846 | -1.151 | -0.6846 |
| 0.6 | 0.4916 | 0.6971 | 0.8802 | 1.215 | 0.6971 | -0.6971 | -1.215 | -0.6971 |
| 0.8 | 0.4978 | 0.7169 | 0.9254 | 1.352 | 0.7169 | -0.7169 | -1.352 | -0.7169 |
| 0.9 | 0.5018 | 0.7308 | 0.9613 | 1.507 | 0.7308 | -0.7308 | -1.507 | -0.7308 |
| 0.99 | 0.506 | 0.7465 | 1.009 | 2.066 | 0.7465 | -0.7465 | -2.066 | -0.7465 |
| 0.999 | 0.5064 | 0.7482 | 1.015 | 2.64 | 0.7482 | -0.7482 | -2.64 | -0.7482 |
| 1 | 0.5065 | 0.7484 | 1.015 | ∞ | 0.7484 | -0.7484 | -∞ | -0.7484 |
The xy plots of the incomplete integrals of the third kind for characteristic n=-1 are depicted in the following figure.
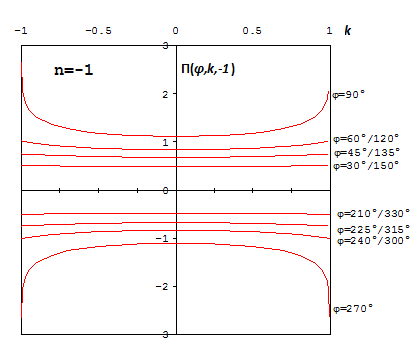
In the following table the values of the incomplete elliptic integral of the third kind are shown for n=5 and a range of k and φ values:
| k | Π(30°,k,5) | Π(45°,k,5) | Π(60°,k,5) | Π(90°,k,5) | Π(135°,k,5) | Π(225°,k,5) | Π(270°,k,5) | Π(315°,k,5) |
|---|---|---|---|---|---|---|---|---|
| -1 | 0.6697 | 0.1964 | -0.009809 | ∞ | 0.1964 | -0.1964 | -∞ | -0.1964 |
| -0.999 | 0.6697 | 0.1966 | -0.00921 | -0.8537 | 0.1966 | -0.1966 | 0.8537 | -0.1966 |
| -0.99 | 0.6695 | 0.1987 | -0.003963 | -0.5619 | 0.1987 | -0.1987 | 0.5619 | -0.1987 |
| -0.9 | 0.6681 | 0.217 | 0.038 | -0.2554 | 0.217 | -0.217 | 0.2554 | -0.217 |
| -0.8 | 0.6663 | 0.2325 | 0.07019 | -0.16 | 0.2325 | -0.2325 | 0.16 | -0.2325 |
| -0.6 | 0.6631 | 0.2536 | 0.1106 | -0.07043 | 0.2536 | -0.2536 | 0.07043 | -0.2536 |
| -0.4 | 0.6606 | 0.266 | 0.1332 | -0.02748 | 0.266 | -0.266 | 0.02748 | -0.266 |
| -0.2 | 0.659 | 0.2726 | 0.1449 | -0.006418 | 0.2726 | -0.2726 | 0.006418 | -0.2726 |
| 0 | 0.6585 | 0.2747 | 0.1486 | 0 | 0.2747 | -0.2747 | 0 | -0.2747 |
| 0.2 | 0.659 | 0.2726 | 0.1449 | -0.006418 | 0.2726 | -0.2726 | 0.006418 | -0.2726 |
| 0.4 | 0.6606 | 0.266 | 0.1332 | -0.02748 | 0.266 | -0.266 | 0.02748 | -0.266 |
| 0.6 | 0.6631 | 0.2536 | 0.1106 | -0.07043 | 0.2536 | -0.2536 | 0.07043 | -0.2536 |
| 0.8 | 0.6663 | 0.2325 | 0.07019 | -0.16 | 0.2325 | -0.2325 | 0.16 | -0.2325 |
| 0.9 | 0.6681 | 0.217 | 0.038 | -0.2554 | 0.217 | -0.217 | 0.2554 | -0.217 |
| 0.99 | 0.6695 | 0.1987 | -0.003963 | -0.5619 | 0.1987 | -0.1987 | 0.5619 | -0.1987 |
| 0.999 | 0.6697 | 0.1966 | -0.00921 | -0.8537 | 0.1966 | -0.1966 | 0.8537 | -0.1966 |
| 1 | 0.6697 | 0.1964 | -0.009809 | ∞ | 0.1964 | -0.1964 | -∞ | -0.1964 |
The xy plots of the incomplete integrals of the third kind for characteristic n=5 are depicted in the following figure.
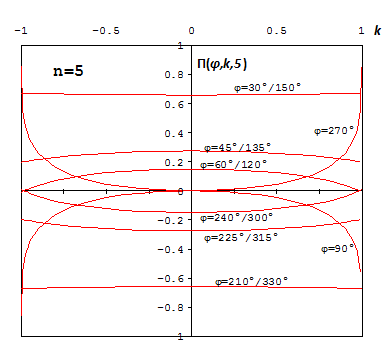
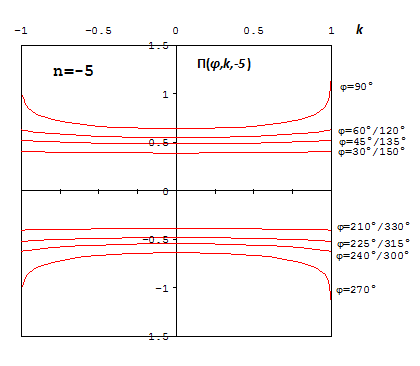
In the following table the values of the incomplete elliptic integral of the third kind are shown for n=-5 and a range of k and φ values:
| k | Π(30°,k,-5) | Π(45°,k,-5) | Π(60°,k,-5) | Π(90°,k,-5) | Π(135°,k,-5) | Π(225°,k,-5) | Π(270°,k,-5) | Π(315°,k,-5) |
|---|---|---|---|---|---|---|---|---|
| -1 | 0.405 | 0.5221 | 0.6272 | ∞ | 0.5221 | -0.5221 | -∞ | -0.5221 |
| -0.999 | 0.405 | 0.522 | 0.627 | 1.177 | 0.522 | -0.522 | -1.177 | -0.522 |
| -0.99 | 0.4047 | 0.5211 | 0.6244 | 0.9849 | 0.5211 | -0.5211 | -0.9849 | -0.5211 |
| -0.9 | 0.4019 | 0.5129 | 0.6038 | 0.7913 | 0.5129 | -0.5129 | -0.7913 | -0.5129 |
| -0.8 | 0.3992 | 0.5055 | 0.5878 | 0.7346 | 0.5055 | -0.5055 | -0.7346 | -0.5055 |
| -0.6 | 0.395 | 0.4948 | 0.5672 | 0.6824 | 0.4948 | -0.4948 | -0.6824 | -0.4948 |
| -0.4 | 0.3922 | 0.488 | 0.5552 | 0.6574 | 0.488 | -0.488 | -0.6574 | -0.488 |
| -0.2 | 0.3905 | 0.4843 | 0.5488 | 0.6451 | 0.4843 | -0.4843 | -0.6451 | -0.4843 |
| 0 | 0.39 | 0.483 | 0.5468 | 0.6413 | 0.483 | -0.483 | -0.6413 | -0.483 |
| 0.2 | 0.3905 | 0.4843 | 0.5488 | 0.6451 | 0.4843 | -0.4843 | -0.6451 | -0.4843 |
| 0.4 | 0.3922 | 0.488 | 0.5552 | 0.6574 | 0.488 | -0.488 | -0.6574 | -0.488 |
| 0.6 | 0.395 | 0.4948 | 0.5672 | 0.6824 | 0.4948 | -0.4948 | -0.6824 | -0.4948 |
| 0.8 | 0.3992 | 0.5055 | 0.5878 | 0.7346 | 0.5055 | -0.5055 | -0.7346 | -0.5055 |
| 0.9 | 0.4019 | 0.5129 | 0.6038 | 0.7913 | 0.5129 | -0.5129 | -0.7913 | -0.5129 |
| 0.99 | 0.4047 | 0.5211 | 0.6244 | 0.9849 | 0.5211 | -0.5211 | -0.9849 | -0.5211 |
| 0.999 | 0.405 | 0.522 | 0.627 | 1.177 | 0.522 | -0.522 | -1.177 | -0.522 |
| 1 | 0.405 | 0.5221 | 0.6272 | ∞ | 0.5221 | -0.5221 | -∞ | -0.5221 |
The xy plots of the incomplete integrals of the third kind for characteristic n=-5 are depicted in the following figure.
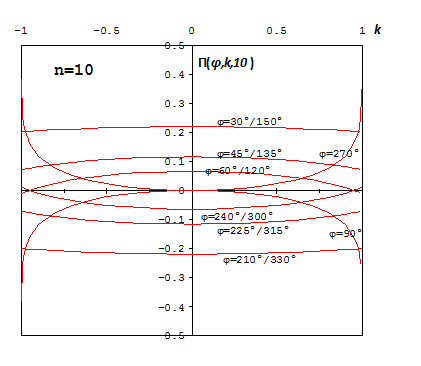
In the following table the values of the incomplete elliptic integral of the third kind are shown for n=10 and a range of k and φ values:
| k | Π(30°,k,10) | Π(45°,k,10) | Π(60°,k,10) | Π(90°,k,10) | Π(135°,k,10) | Π(225°,k,10) | Π(270°,k,10) | Π(315°,k,10) |
|---|---|---|---|---|---|---|---|---|
| -1 | 0.2009 | 0.07115 | -0.01182 | ∞ | 0.07115 | -0.07115 | -∞ | -0.07115 |
| -0.999 | 0.201 | 0.07127 | -0.01156 | -0.384 | 0.07127 | -0.07127 | 0.384 | -0.07127 |
| -0.99 | 0.2014 | 0.07233 | -0.009216 | -0.2545 | 0.07233 | -0.07233 | 0.2545 | -0.07233 |
| -0.9 | 0.205 | 0.08177 | 0.009852 | -0.1187 | 0.08177 | -0.08177 | 0.1187 | -0.08177 |
| -0.8 | 0.2085 | 0.0902 | 0.02502 | -0.07571 | 0.0902 | -0.0902 | 0.07571 | -0.0902 |
| -0.6 | 0.2136 | 0.1023 | 0.04497 | -0.03421 | 0.1023 | -0.1023 | 0.03421 | -0.1023 |
| -0.4 | 0.217 | 0.11 | 0.05669 | -0.01357 | 0.11 | -0.11 | 0.01357 | -0.11 |
| -0.2 | 0.2189 | 0.1142 | 0.06297 | -0.0032 | 0.1142 | -0.1142 | 0.0032 | -0.1142 |
| 0 | 0.2195 | 0.1155 | 0.06496 | 0 | 0.1155 | -0.1155 | 0 | -0.1155 |
| 0.2 | 0.2189 | 0.1142 | 0.06297 | -0.0032 | 0.1142 | -0.1142 | 0.0032 | -0.1142 |
| 0.4 | 0.217 | 0.11 | 0.05669 | -0.01357 | 0.11 | -0.11 | 0.01357 | -0.11 |
| 0.6 | 0.2136 | 0.1023 | 0.04497 | -0.03421 | 0.1023 | -0.1023 | 0.03421 | -0.1023 |
| 0.8 | 0.2085 | 0.0902 | 0.02502 | -0.07571 | 0.0902 | -0.0902 | 0.07571 | -0.0902 |
| 0.9 | 0.205 | 0.08177 | 0.009852 | -0.1187 | 0.08177 | -0.08177 | 0.1187 | -0.08177 |
| 0.99 | 0.2014 | 0.07233 | -0.009216 | -0.2545 | 0.07233 | -0.07233 | 0.2545 | -0.07233 |
| 0.999 | 0.201 | 0.07127 | -0.01156 | -0.384 | 0.07127 | -0.07127 | 0.384 | -0.07127 |
| 1 | 0.2009 | 0.07115 | -0.01182 | ∞ | 0.07115 | -0.07115 | -∞ | -0.07115 |
The xy plots of the incomplete integrals of the third kind for characteristic n=10 are depicted in the following figure.
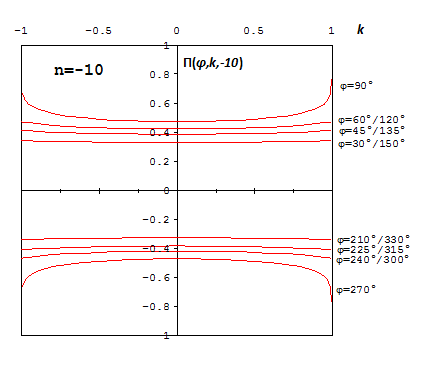
In the following table the values of the incomplete elliptic integral of the third kind are shown for n=-10 and a range of k and φ values:
| k | Π(30°,k,-10) | Π(45°,k,-10) | Π(60°,k,-10) | Π(90°,k,-10) | Π(135°,k,-10) | Π(225°,k,-10) | Π(270°,k,-10) | Π(315°,k,-10) |
|---|---|---|---|---|---|---|---|---|
| -1 | 0.3394 | 0.4108 | 0.4706 | ∞ | 0.4108 | -0.4108 | -∞ | -0.4108 |
| -0.999 | 0.3394 | 0.4107 | 0.4705 | 0.7719 | 0.4107 | -0.4107 | -0.7719 | -0.4107 |
| -0.99 | 0.3391 | 0.4101 | 0.4689 | 0.6666 | 0.4101 | -0.4101 | -0.6666 | -0.4101 |
| -0.9 | 0.3371 | 0.4048 | 0.4566 | 0.5597 | 0.4048 | -0.4048 | -0.5597 | -0.4048 |
| -0.8 | 0.3352 | 0.4001 | 0.447 | 0.5276 | 0.4001 | -0.4001 | -0.5276 | -0.4001 |
| -0.6 | 0.3321 | 0.3931 | 0.4343 | 0.4977 | 0.3931 | -0.3931 | -0.4977 | -0.3931 |
| -0.4 | 0.3301 | 0.3886 | 0.4269 | 0.4831 | 0.3886 | -0.3886 | -0.4831 | -0.3886 |
| -0.2 | 0.3289 | 0.3861 | 0.4229 | 0.4758 | 0.3861 | -0.3861 | -0.4758 | -0.3861 |
| 0 | 0.3285 | 0.3853 | 0.4216 | 0.4736 | 0.3853 | -0.3853 | -0.4736 | -0.3853 |
| 0.2 | 0.3289 | 0.3861 | 0.4229 | 0.4758 | 0.3861 | -0.3861 | -0.4758 | -0.3861 |
| 0.4 | 0.3301 | 0.3886 | 0.4269 | 0.4831 | 0.3886 | -0.3886 | -0.4831 | -0.3886 |
| 0.6 | 0.3321 | 0.3931 | 0.4343 | 0.4977 | 0.3931 | -0.3931 | -0.4977 | -0.3931 |
| 0.8 | 0.3352 | 0.4001 | 0.447 | 0.5276 | 0.4001 | -0.4001 | -0.5276 | -0.4001 |
| 0.9 | 0.3371 | 0.4048 | 0.4566 | 0.5597 | 0.4048 | -0.4048 | -0.5597 | -0.4048 |
| 0.99 | 0.3391 | 0.4101 | 0.4689 | 0.6666 | 0.4101 | -0.4101 | -0.6666 | -0.4101 |
| 0.999 | 0.3394 | 0.4107 | 0.4705 | 0.7719 | 0.4107 | -0.4107 | -0.7719 | -0.4107 |
| 1 | 0.3394 | 0.4108 | 0.4706 | ∞ | 0.4108 | -0.4108 | -∞ | -0.4108 |
The xy plots of the incomplete integrals of the third kind for characteristic n=-10 are depicted in the following figure.